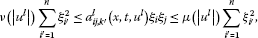- Research
- Open access
- Published:
Diffraction problems for quasilinear parabolic systems with boundary intersecting interfaces
Boundary Value Problems volume 2013, Article number: 99 (2013)
Abstract
In this paper, we discuss the n-dimensional diffraction problem for weakly coupled quasilinear parabolic system on a bounded domain Ω, where the interfaces () are allowed to intersect with the outer boundary ∂ Ω and the coefficients of the equations are allowed to be discontinuous on the interfaces. The aim is to show the existence of solutions by approximation method. The approximation problem is a diffraction problem with interfaces, which do not intersect with ∂ Ω.
MSC:35R05, 35K57, 35K65.
1 Introduction
Let Ω be a bounded domain in with boundary ∂ Ω (), and let Ω be partitioned into a finite number of subdomains () separated by , where , , are interfaces, which do not intersect with each other. For any , set
In this paper, we consider the diffraction problem for quasilinear parabolic reaction-diffusion system in the form
where , , , , ,
repeated indices i or j indicate summation from 1 to n, is the unit normal vector to Γ (the positive direction of is fixed in advance), the symbol denotes the jump of a quantity across , and the coefficients , and are allowed to be discontinuous on . In the following, we refer to the conditions on in (1.1) as diffraction conditions.
The diffraction problems often appear in different fields of physics, ecology, and technics. In some of them, the interfaces are allowed to intersect with the outer boundary ∂ Ω (see [1–5]). The linear diffraction problems have been treated by many researchers (see [1–10]). For the quasilinear parabolic and elliptic diffraction problems, when all of the interfaces do not intersect with ∂ Ω, the existence and uniqueness of the solutions have been investigated in [11–14] by Leray-Schauder principle and the method of upper and lower solutions. In this paper, we investigate the existence of solutions of (1.1) when the interfaces are allowed to intersect with ∂ Ω. In this case, because of the existence of the intersection of Γ and ∂ Ω, the methods in [11–14] can not be extended. We shall show the existence of solutions by approximation method. The approximation problem is a diffraction problem with interfaces which do not intersect with ∂ Ω.
The plan of the paper is as follows. In Sect. 2, we give the notations, hypotheses and an example, and state the existence theorem of the solutions. Section 3 is devoted to the proof of the existence theorem.
2 The hypotheses, main result and example
2.1 The notations, hypotheses and main result
First, let us introduce more notations and function spaces.
For any set S, denotes its closure. The symbol means that and .
Let
where , , intersect with the outer boundary ∂ Ω, and , do not intersect with ∂ Ω. Assume that the domain Ω is partitioned into subdomains , , separated by interfaces , and partitioned into , , separated by . The interface of and is . Then . Set
We see that .
is the spaces of Hölder continuous in with exponent . and are the Hilbert spaces with scalar products and , respectively. Let
For the vector functions with N-components we denote the above function spaces by , , , and , respectively.
Moreover, we recall the following.
Write u in the split form
The vector function is said to be mixed quasimonotone in with index vector if for each , there exist nonnegative integers , , satisfying
such that is nondecreasing in , and is nonincreasing in for all .
The following hypotheses will be used in this paper:
-
(H)
(i) ∂ Ω and , , are of for some exponent and there exist and such that for every open ball centered at and radius ,
Assume that for each ,
and
-
(ii)
Assume that
(2.2)
where and are defined on , are defined on , and all of them are allowed to be discontinuous on .
-
(iii)
There exist constant vectors and , , such that
(2.3)
where , are all independent of . Let
The vector functions , , are mixed quasimonotone in with the same index vector .
-
(iv)
For each , , , , (), . There exist a positive nonincreasing function and a positive nondecreasing function for such that
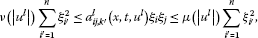 (2.4)
(2.4)

For each , for some domain Ξ with , (), and the following compatibility condition on holds:
Definition 2.2 A function u is said to be a solution of (1.1) if u possesses the following properties: (i) For some , , . For any given and , there exists , , such that and , , ; (ii) u satisfies the equations in (1.1) for , , the diffraction conditions for and the parabolic boundary conditions for .
The main result in this paper is the following existence theorem.
Theorem 2.1 Let Hypothesis (H) hold. Then problem (1.1) has a solution u in .
2.2 An example
We next give an example satisfying the conditions in Hypothesis (H).
Example 2.1 In problem (1.1), let
where and , and let
The outer boundary of domain is a circle of radius 10 with the center at the origin, whereas the interface curves are two parabolas and a smaller circle of radius 1 (see Figure 1). We see that and intersect with ∂ Ω, and does not.
For the coefficients of the equations and the boundary values in (1.1) we set
where
with , and , , , and are all positive constants for , .
Then
For each , let
We find that these functions satisfy (2.2) and the hypothesis (iv) of (H). Set . Then the requirements on M in (2.3) become
It follows from these inequalities that there exist positive constant vector M, such that m and M satisfy (2.3). Furthermore, the vector functions , , are mixed quasimonotone in with the same index vector . The above arguments show that the conditions in Hypothesis (H) can be satisfied.
3 The proof of the existence theorem
3.1 Preliminaries
Lemma 3.1 The following statements hold true:
-
(i)
For any given , if for some , then
-
(ii)
There exists a positive number such that for any given , if , then
Proof By (2.1), if and , then . Thus for each , . Again by (2.1) we get . This proves the result in (i).
For any given , if and , then it follows from (i) that for all . Since , there exist positive constants such that
Hence, the conclusion in (ii) follows from the above relation by taking . □
For an arbitrary ε, , let be smooth function with values between 0 and 1 such that for all , for and for . Define
Lemma 3.2 , , are smooth functions with values between 0 and 1, and possess the property
Let functions , , be defined on , and let
Then for any ,
Proof Since (3.2) is a special case of (3.4) with for all , we only prove (3.4).
Case 1. If , then the conclusion of (i) in Lemma 3.1 implies that and for all . (3.1) yields that and for . These, together with (3.3), imply that .
Case 2. If , then the conclusion of (ii) in Lemma 3.1 shows that and for all . Hence, and for all . Again by (3.3) we get .
Case 3. If and for some , then Lemma 3.1 yields that , for all , and that , for all . Hence, and for . Therefore, .
Case 4. If for some , then it follows from Lemma 3.1 that and for all , and that . Again by the conclusion of (i) in Lemma 3.1 we have and for all . Hence, , and for . Thus, . □
3.2 The approximation problem of (1.1)
In this subsection, we construct a problem to approximate (1.1).
For each , let
It follows from hypothesis (iv) of (H), (3.2) and (3.5) that , are in (), is in (), the vector function is mixed quasimonotone in with index vector , and


We note that the functions , and are continuous on , and are allowed to be discontinuous on .
For each , there exists such that . Take two subdomains , satisfying . Let be an arbitrary smooth function taking values in such that for and for . Set
with a sufficiently smooth nonnegative averaging kernel that is equal to zero for and is such that . Then from the hypothesis (iv) of (H) and [[1], Chapter II] we know that for each , is in , is in (), in and in . Thus,
where is a positive constant, independent of ε. Furthermore, (3.4), (3.5) and (3.8) show that for small enough ε,
These, together with (2.6), imply that
For any given ε, , consider the approximation diffraction problem of (1.1)
where
We note that the interfaces in (3.11) are () which do not intersect with ∂ Ω. In view of (3.10), the compatibility condition on holds.
Proposition 3.1 Problem (3.11) has a unique piecewise classical solution in possessing the following properties:
Proof Problem (3.11) is a special case of [[13], problem (1.1)] without time delays. Formulas (2.3) and (3.5) show that , are a pair of bounded and coupled weak upper and lower solutions of (3.11) in the sense of [[13], Definition 2.2]. We find that the conditions of [[13], Theorem 4.1] are all fulfilled. Then from [[13], Theorem 4.1], we obtain that problem (3.11) has a unique piecewise classical solution in possessing the properties in (3.12). □
3.3 The uniform estimates of
In the following discussion, let be an arbitrary open ball of radius ρ with center at , and let be an arbitrary cylinder of the form .
For each , consider the equality for any function from with and for any , t from . In view of , it follows from (3.6), (3.7), (3.9) and the formula of integration by parts that


Similarly, for any and for every we get
Lemma 3.3 There exist constants () and C depending only on (), , , , , and , independent of ε, such that


Proof (3.16) follows from (3.14), (3.6), (3.7), (3.9) and [[1], Chapter V, Theorem 1.1 and Remark 1.2]. Setting in (3.14) and using Cauchy’s inequality, we can obtain (3.17). □
Lemma 3.4 For any given , let and . Then there exist positive constants () and depending only on (), and the parameters , , , , , and , independent of ε, such that for any satisfying ,


For any given , let and . Then there exist positive constants () and depending only on (), and the parameters , , , , , and , such that
Proof Choose a subdomain B satisfying . (3.4) and (3.5) show that for small enough ε,
Then the same proofs as those of [[13], formulas (3.30) and (3.31)] give (3.18) and (3.19). If , then for some , . Hence, the conclusion in (3.20) follows from (3.18), (3.19), (3.21) and the same argument as that for [[13], formula (3.37)]. □
In the rest of this subsection, let be an arbitrary fixed number in , and let be an arbitrary fixed subdomain satisfying , and . We next investigate the uniform estimates in the neighborhood of . Let be any point of . [[2], Chapter 3, Section 16] and [13] show that there exists a ball with center at such that we can straighten out by introducing a local coordinate system . Our assumptions concerning Γ imply that we can divide into a finite number of pieces and to introduce for each of them coordinates y. Since the investigations in the rest of this subsection are local properties, we can assume without loss of generality that the interface lies in the plane . Then by (3.4), when the coefficients of problem (3.11) can be represented in the form
and the diffraction conditions on in problem (1.1) can be represented in the form
Lemma 3.5 Let . Then there exist positive constants () and depending only on (), , and the parameters , , , , , and , independent of ε, such that



Proof It follows from (3.22) and Hypothesis (H) that

and from the equations in (3.11) that
Then using (3.13), (3.15), (3.22), (3.27) and (3.28), we can prove (3.24)-(3.26) by a slight modification of the proofs of [[13], formulas (3.30) and (3.31)]. The detailed proofs are omitted. □
3.4 The proof of Theorem 2.1
From estimates (3.16), (3.17) and the Arzela-Ascoli theorem it follows that we can find a subsequence (we retain the same notation for it) such that converges in to u and converges weakly in to for each . Then and . Furthermore, the parabolic boundary conditions for in (3.11) imply that u satisfies the parabolic boundary conditions in (1.1).
For any given , and for any , , (3.20) yields that there exists a subsequence (denoted by still) such that converges in to u. By letting , from (3.21) and the equations in (3.11) we get that
Since and are arbitrary, then u satisfies the equations in (3.11) for .
For any given and for any , , we see from (3.18), (3.19) that there exists a subsequence (denoted by still) such that for each and for any satisfying , converges in to , and converges in to . Hence
By letting we conclude from (3.21) and the diffraction conditions on for in (3.11) that
For any given and satisfying , and , the estimates (3.24)-(3.26) imply that for any given there exists a subsequence (denoted by still) such that for each , ,
Then
We next show that . For any ,
By (3.27), (3.17), we get
and by (2.2), (3.22),
Since converges weakly in to for each , then as . Hence, converges weakly in to for each . This, together with (3.31), implies that
and u satisfies the diffraction conditions on in (3.23).
In view of (3.30) u satisfies the diffraction conditions on in (1.1). Furthermore, (3.29), (3.32) and (3.33) imply that for any , ,
for some . Therefore, u is a solution of (1.1). This completes the proof of Theorem 2.1.
References
Ladyzenskaya OA, Solonnikov VA, Ural’ceva NN: Linear and Quasilinear Equations of Parabolic Type. Am. Math. Soc., Providence; 1968.
Ladyzenskaya OA, Ural’ceva NN: Linear and Quasilinear Elliptic Equations. Academic Press, New York; 1968.
Ladyzenskaya OA, Ryvkind VJ, Ural’ceva NN: Solvability of diffraction problems in the classical sense. Tr. Mat. Inst. Steklova 1966, 92: 116-146. in Russian
Yi FH: Global classical solution of Muskat free boundary problem. J. Math. Anal. Appl. 2003, 288: 442-461. 10.1016/j.jmaa.2003.09.003
Druet, PE: Global Lipschitz continuity for elliptic transmission problems with a boundary intersecting interface. Weierstrass Institute for Applied Analysis and Stochastics, WIAS Preprint No. 1571 (2010)
Kamynin LI: On the linear Verigin problem. Dokl. Akad. Nauk SSSR 1963, 150: 1210-1213. in Russian
Kamynin LI: The method of heat potentials for a parabolic equation with discontinuous coefficients. Sib. Mat. Zh. 1963, 4: 1071-1105. in Russian
Chen Z, Zou Z: Finite element methods and their convergence for elliptic and parabolic interface problems. Numer. Math. 1998, 79: 175-202. 10.1007/s002110050336
Huang J, Zou J: Some new a priori estimates for second-order elliptic and parabolic interface problems. J. Differ. Equ. 2002, 184: 570-586. 10.1006/jdeq.2001.4154
Evans LC: A free boundary problem: the flow of two immiscible fluids in a one-dimmensional porous medium. I. Indiana Univ. Math. J. 1977, 26: 914-932.
Rivkind V, Ural’tseva N: Classical solvability and linear schemas for the approximated solutions of the diffraction problem for quasilinear elliptic and parabolic equations. Mat. Zametki 1972, 3: 69-111. in Russian
Boyadjiev G, Kutev N: Diffraction problems for quasilinear reaction-diffusion systems. Nonlinear Anal. 2003, 55: 905-926. 10.1016/j.na.2003.08.006
Tan QJ: Systems of quasilinear parabolic equations with discontinuous coefficients and continuous delays. Adv. Differ. Equ. 2011., 2011: Article ID 925173. doi:10.1155/2011/925173
Tan QJ, Leng ZJ: The method of upper and lower solutions for diffraction problems of quasilinear elliptic reaction-diffusion systems. J. Math. Anal. Appl. 2011, 380: 363-376. 10.1016/j.jmaa.2011.02.050
Pao CV: Nonlinear Parabolic and Elliptic Equations. Plenum Press, New York; 1992.
Acknowledgements
Dedicated to Professor Hari M Srivastava.
The authors would like to thank the reviewers and the editors for their valuable suggestions and comments. The work was supported by the research fund of Department of Education of Sichuan Province (10ZC127) and the research fund of Chengdu Normal University (CSYXM12-06).
Author information
Authors and Affiliations
Corresponding author
Additional information
Competing interests
The authors declare that they have no competing interests.
Authors’ contributions
All authors read and approved the final manuscript.
Authors’ original submitted files for images
Below are the links to the authors’ original submitted files for images.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Tan, QJ., Pan, CY. Diffraction problems for quasilinear parabolic systems with boundary intersecting interfaces. Bound Value Probl 2013, 99 (2013). https://doi.org/10.1186/1687-2770-2013-99
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/1687-2770-2013-99